Minimum variance portfolio

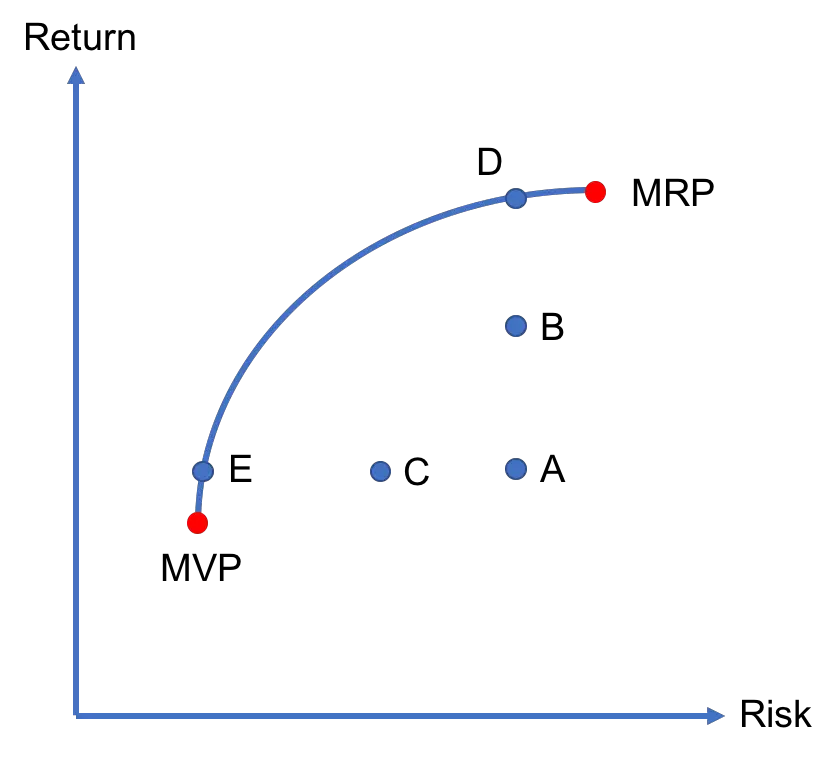
In a market with multiple risky assets, the minimum variance portfolio is a particular combination of those assets that yields the minimum volatility. To be more specific, consider the market depicted in Figure 1. Here, the blue curve represents the efficient frontier. That is, all portfolios that lie on it are efficient portfolios (e.g., D and E) whereas those that lie below it are inefficient portfolios (e.g., A, B, and C). In this context, the leftmost portfolio on the efficient frontier is the minimum variance portfolio (MVP). This is because it is the efficient portfolio that has the lowest level of risk. The MVP appeals to investors that are particularly risk-averse.

Minimum variance portfolio formula

Let’s suppose we have two risky assets: A and B. Then, we can write the portfolio variance as:

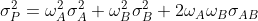
where σ_A and σ_B are the standard deviations of returns (i.e., return volatility) for these two assets, σ_AB is the covariance of returns, and ω_A and ω_B are the investment weights. Given that ω_A + ω_B = 1 (i.e., we’re fully invested in these two assets), we have ω_B = 1 − ω_A. So, we can rewrite the portfolio variance as follows:

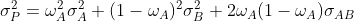
We’d like to find the investment weight ω_A* that yields the minimum variance portfolio:

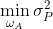
We can find that by taking the derivative of portfolio variance with respect to ω_A and setting it equal to zero:

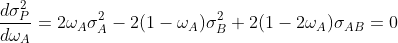
Solving for the optimal investment weight ω_A* yields the minimum variance portfolio formula:

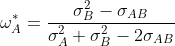
Remember that the covariance between the returns of two assets σ_AB can also be written as ρ_ABσ_Aσ_B, where ρ_AB is the correlation coefficient. So, we can write the formula using the correlation coefficient instead of covariance as well:

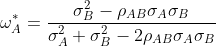
Minimum variance portfolio calculator

Three inputs are needed to use our minimum variance portfolio calculator:

• The standard deviation of returns on the 1st asset in percentages (σ_A ≥ 0).
• The standard deviation of returns on the 2nd asset in percentages (σ_B ≥ 0).
• The correlation coefficient between the returns of the two assets (ρ_AB), which needs to be between −1 and +1.

Note that if the covariance between the returns of the two assets (σ_AB) is known/given instead of the correlation, the latter can be easily calculated as: ρ_AB = σ_AB / (σ_Aσ_B).

Once the inputs are entered, the calculator gives the optimal investment weight for each asset (ω_A* and ω_B*), and the variance (σ_MVP²) and standard deviation (σ_MVP) of the portfolio that has the minimum variance. It is worth highlighting that either ω_A* or ω_B* can be negative, in which case short selling is implied.

To illustrate the use of the calculator, try the following example. If asset A has a volatility of 20%, asset B has a volatility of 10%, and the correlation coefficient between the returns of A and B is −0.25, then investing 25% in A and 75% in B yields the minimum variance portfolio, which has a variance of 62.5 and volatility of 7.9%.

Summary

One of the most significant insights of modern portfolio theory is that investors can reduce risk through diversification. In this lesson, we explained the concept of the (global) minimum variance portfolio. For any given number of assets, this portfolio has the lowest risk where risk is measured in terms of variance.

Further reading:

Clarke, de Silva, and Thorley (2006), “Minimum-Variance Portfolios in the U.S. Equity Market“, The Journal of Portfolio Management, Vol. 33(1), pp. 10-24.

What is next?

This lesson is part of our free course on investments.

• Next lesson: We will show how to obtain a capital allocation line by combining the risk-free asset with a risky asset.
• Previous lesson: We discussed what is meant by an efficient frontier, distinguishing between efficient portfolios and inefficient ones.

If you’ve got any feedback for us, you can reach us here. And, if you enjoy reading our content, you can help us by spreading the word on social media and within your social networks.